This article is one in a long series which I hope will help explain the ins and outs of DFA – Dimensional Fund Advisors. NOTE: This is my interpretation and explanation only. For the final word, please refer to the DFA Canada Website.
Introduction To The Fama-French Three Factor Model
First, as a refresher let’s look at the CAPM. Remember, this asset pricing model (the “single” factor model) says that an investor’s expected return is based on their exposure to the market factor (or Beta). Here is the formula again:
E(Rp) = Rf + β(Rm – Rf)
As I alluded to in the last part of this series, the Fama-French Three Factor Model (FFTFM henceforth) uses three factors to explain expected return: 1) Market Factor, 2) Size Factor, and 3) Value Factor. Just as CAPM includes a measure of the amount of exposure to the market factor, the FFTFM includes measures of the exposure to each of the three factors.
The Fama-French Three Factor Model:
E(Rp) = Rf + β(Rm – Rf) + s(Small – Big) + h(High BtM – Low BtM)
(Small – Big) and (High BtM – Low BtM) are analogous to (Rm – Rf). Remember that (Rm – Rf) is the equity premium or market factor – it is just the excess return of stocks over t-bills. Similarly (Small – Big) is the excess return of Small stocks versus Big stocks, and (High BtM – Low BtM) is the excess return of Value stocks over Growth stocks. You can think of each as having their own Beta, except it’s not called Beta for each – it’s only called Beta for measuring the sensitivity to the market factor. There is not really a name for the “other” Betas, they are just represented as “s” and “h” – each is just a measure of the amount of exposure to the other two factors (size and value).
In fact, the way the formula is normally written uses SMB to represent “Small Minus Big” and HML to represent “High BtM Minus Low BtM”. Therefore you will see the formula written as follows (but it means the same as above):
E(Rp) = Rf + β(Rm – Rf) + s(SMB) + h(HML)
And, just to bash you over the head again with the same old thing, in plain english this is saying that the investor’s expected return is equal to the risk-free rate PLUS their exposure to the market factor PLUS their exposure to the size factor PLUS their exposure to the value factor.
Okay Preet, So What?
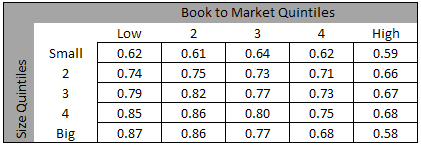
Well I suppose the best way to explain why this three factor model has garnered so much attention is to look at how Fama and French looked at it. They took the total stock market and they chopped it up into a 5 x 5 matrix (Book to Market quintiles by Size quintiles) as follows:
It is also important to know what R² is – according to Wikipedia: “the coefficient of determination, R2, is the proportion of variability in a data set that is accounted for by a statistical model… R2 is a statistic that will give some information about the goodness of fit of a model. In regression, the R2 coefficient of determination is a statistical measure of how well the regression line approximates the real data points. An R2 of 1.0 indicates that the regression line perfectly fits the data.” Basically the closer to 1.0 the R² is, the more “explanatory power” of a model.
If you look at the CAPM regressions for “monthly value-weight portfolio returns from July 1963 to December 2007” for a total of 534 months of data (information sourced from Eugene Fama and DFA) then the R² values for this 5×5 cross-section of the total market looks as follows (remember, this is for the CAPM):
So what is being said here is that for each slice of the 25 slices of the market based on differing size and value factors, the CAPM really only does a half decent job for larger stocks with low Book to Market values – coincidentally, the S&P 500 has many large cap growth stocks. But once we stray away from this, the CAPM does an increasingly poorer job of explaining the variation in returns. Of course, this begs the question: what about the Three Factor regressions?
Here are the “Three Factor Regressions for Monthly Value-Weight Portfolio Returns from July 1963 to December 2007”, again a total of 534 months of data and this was sourced from Eugene Fama and DFA, and again looking at the R² values:
I’ll stop there for today, but Part XI will address some of the early criticisms of this model. Part XII will look at how widespread this model has become, even though you many not realize it.


It’s not surprising that the coefficient of determination is better with the Fama-French model because they introduce extra independent variables. Using s=h=0, Fama-French reduces to CAPM. But, the data has been mined for the values of s and h that maximize the coefficient of determination. So, Fama-French has to beat CAPM. The fact that it beats CAPM by so much means that they may be on to something with their approach.
A interesting test would be to add the following term to CAPM: u(high v – low v), where v is the number of vowels in the company’s name. If this improves the coefficient of determination significantly after doing the regression to find the best constant u, then we might think twice about being impressed by Fama-French.
I’d be interested in seeing what the effect is on CAPM’s coefficient of determination if we replace (Rm-Rf) with (Rm-Rf-c), where c represents a constant gap above the T-bill rate to take into account the fact that we can’t borrow at the T-bill rate. The regression would give the optimal value for c, and we could tell from the resulting coefficients of determination whether this is a significantly better model.
When the original F/F paper was published in 1992 a lot of people assumed it was nothing more than “data mining” – and without going into much detail F/F then looked at another 36 years of US data: found the same thing. They looked to other markets from around the world: found the same thing. They silenced the criticisms of data mining years ago.
Preet, you are doing an excellent job in explaining this information – why are you wasting your time as an advisor? I can’t imagine the average investor will ever grasp this and have the conviction to stick it out.
For the other commenter, I cannot tell if you agree or disagree with F/F’s research and conclusions based on your comments, but if you just supply your spreadsheet with an artificially larger Rf you could account for a greater cost of borrowing and a simultaneous decrease in the market factor, and you will get the answer of ‘it still works brilliantly’ for c < about 1, and ‘it still works really well’ for c < 2.
The idea of trying to find a vowel factor would be pure data mining and if you found it in one set of data you wouldn’t find it in any others.
SD: The reason you can’t tell whether I agree with Fama and French is because I haven’t made up my mind yet. You’re right that the vowel factor has to be pure data mining. That was the idea. Adding the vowel factor would give us an idea of how much the coefficient of determination increases when adding an extra independent variable for the regression. The Fama-French model gets a boost from having two extra independent variables. The question is whether their results are even better than one would expect from adding two pointless variables like the vowel thing.
I didn’t understand your comments about the greater cost of borrowing. Do you mean “c < 1%” and “c < 2%”? Are you saying that you have an idea how these values would fare compared to pure CAPM after doing the calculations?
@Michael James – I suppose it couldn’t hurt to ask Fama or French for the results you are looking for with regards to subtracting the spread between Rf and c – it *should* just be a matter of plugging it in and getting an answer.
@SD – Thanks for the note. You’ve stolen part of my next post in which we look at data sets over and above those used in the original paper. But to answer your question about being an advisor: I’m honestly just trying to do what’s right for investors as an advisor, but this blog is not really aimed at clients/potential clients. It’s largely for pleasure and learning (on my part!) Also, I do have a question about your last paragraph as well – do you have the ability to provide the data for what Michael James is looking for? I too would be most interested in seeing that.